In Statistics, the binomial distribution (이항 분포) with parameters n and p is the discrete Probability distribution of the number of successes in a sequence of n independent yes/no experiments, each of which yields success with probability p. (http://en.wikipedia.org/wiki/Binomial_distribution)

Bernoulli trial을 반복하는 경우, 즉 둘중에 한가지 사건이 발생할 event의 이산적 확률분포이다. Bernoulli distribution이라고도 한다.

A라는 사건이 일어날 확률P(A)를 p라 하고, 일어나지 않을 확률을 q=1-p라고 하고, n번 시행했다고 하고, 그중에 A가 일어난횟수를 x라고 하면,

$$ Pr(K=k) = f(k; n, p) = \frac{n!}{k!(n-k)!}p^k(1-p)^{n-k} $$

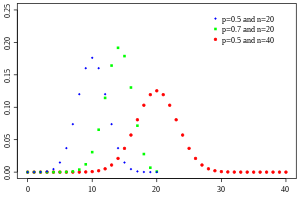
이항분포에서 평균 mu는 np이고, 분산 sigma^2 은 npq 이다. 계산식에 Combination이 사용된다.

이항분포의 극한조건 즉 n --> 무한대, p --> 0 인 경우, Poisson distribution이 된다.

관련정보

• 온라인 게임에서의 이항 분포 2015-05-21

Rosalind 문제 풀이 중 적어도 3번 이상 나올 확률 구하는 문제가 있었는데, 이를 각각 3번, 4번, 5번 각각에 대해 모든 조합의 확률을 구해서 곱했는데 이게 바로 이항 분포 식이였음. --Hyungyong Kim, 2013-06-04

Incoming Links #

Related Articles (Article 0) #

• Maximum likelihood
• Poisson distribution
• Probability distribution

Related Education Events (EducationEvent 1) #

• Hanyang University/맞춤의료학세미나1

Suggested Pages #

• 0.266 Expectation maximization
• 0.121 Normal distribution
• 0.107 Markov chain Monte Carlo
• 0.070 Statistical hypothesis testing
• 0.032 QTL
• 0.029 Statistical inference
• 0.024 Central limit theorem
• 0.023 Bioinformatics
• 0.023 Hyuna Kim
• 0.022 Tennis
• More suggestions...